Question

The driver of a car traveling at 50 ft/sec suddenly applies the brakes. The position of the car is s(t) = 50t - 2t2, t seconds after the driver applies the brakes. How many seconds after the driver applies the brakes does the car come to a stop?

Answer 1

s(t) = 50t - 2t^2
v(t) = 50 - 4t
a(t) = -4

When v(t) = 0, the car stops.
v = u +at
0 = 50 + (-4)t
t=12.5s

Answer 2

The car come to a stop in 12.5 seconds after the driver applies the brakes

Explanation:

Given :The position of the car is
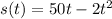`s(t) = 50t - 2t^ 2`, t seconds after the driver applies the brakes.

To Find : How many seconds after the driver applies the brakes does the car come to a stop?

Solution:

`s(t) = 50t - 2t^ 2`

`V =(ds)/(dt)`

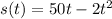
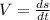
Where V is Speed of car

So,
`V =(d(50t - 2t^ 2))/(dt)`

`V =50-4t`

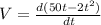
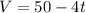
So,Speed of the car is
`V =50-4t`

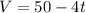
Now we are supposed to find How many seconds after the driver applies the brakes does the car come to a stop.

So, Substitute V = 0

`0 =50-4t`

`4t=50`

`t=(50)/(4)`

`t=12.5`

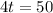
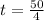
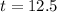
Hence the car come to a stop in 12.5 seconds after the driver applies the brakes