Question

PLEASE HELP!! CALCULUS QUESTION

How to do this?

Answer 1

`\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy = 28ln(7) - 24ln(6) - 4`

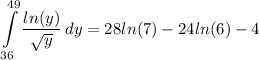
General Formulas and Concepts:

Algebra I

• Exponential Rule [Rewrite]:
  `\displaystyle b^(-m) = (1)/(b^m)`
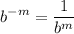
• Exponential Rule [Root Rewrite]:
  `\displaystyle \sqrt[n]{x} = x^{(1)/(n)}`

Calculus

Derivatives

Derivative Notation

Basic Power Rule:

• f(x) = cxⁿ
• f’(x) = c·nxⁿ⁻¹

Logarithmic Derivative:
`\displaystyle (d)/(dx) [lnu] = (u')/(u)`

Integrals

• Definite Integrals

Integration Rule [Reverse Power Rule]:
`\displaystyle \int {x^n} \, dx = (x^(n + 1))/(n + 1) + C`

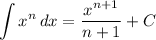
Integration Rule [Fundamental Theorem of Calculus 1]:
`\displaystyle \int\limits^b_a {f(x)} \, dx = F(b) - F(a)`

Integration Property [Multiplied Constant]:
`\displaystyle \int {cf(x)} \, dx = c \int {f(x)} \, dx`

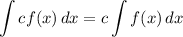
Integration by Parts:
`\displaystyle \int {u} \, dv = uv - \int {v} \, du`

• [IBP] LIPET: Logs, inverses, Polynomials, Exponentials, Trig

Explanation:

Step 1: Define

Identify

`\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy`

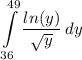
Step 2: Integrate Pt. 1

Identify and find variables for Integration by Parts.

1. [LIPET] Set:
   `\displaystyle u = ln(y)`
2. [LIPET] Set:
   `\displaystyle dv = (1)/(√(y)) \ dy`
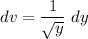
3. [u] Differentiate [Logarithmic Derivative]:
   `\displaystyle (du)/(dy) = (1)/(y)`
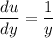
4. [u] Rewrite:
   `\displaystyle du = (1)/(y) \ dy`
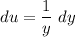
5. [dv] Integrate [Integration Rule - Reverse Power Rule]:
   `\displaystyle v = 2√(y)`

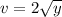
Step 3: Integrate Pt. 2

1. [Integral] Integration by Parts:
   `\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy = \bigg[ 2ln(y)√(y) \bigg] \bigg| \limits^(49)_(36) - \int\limits^(49)_(36) {(2√(y))/(y)} \, dy`
2. [Integral] Simplify/Rewrite [Integration Property - Multiplied Constant]:
   `\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy = \bigg[ 2ln(y)√(y) \bigg] \bigg| \limits^(49)_(36) - 2\int\limits^(49)_(36) {(1)/(√(y))} \, dy`
3. [Integral] Reverse Power Rule:
   `\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy = \bigg[ 2ln(y)√(y) \bigg] \bigg| \limits^(49)_(36) - 2 \bigg[ 2√(y) \bigg] \bigg| \limits^(49)_(36)`
4. Evaluate [Integration Rule - FTC 1]:
   `\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy = 28ln(7) - 24ln(6) - 2(2)`
5. Simplify:
   `\displaystyle \int\limits^(49)_(36) {(ln(y))/(√(y))} \, dy = 28ln(7) - 24ln(6) - 4`

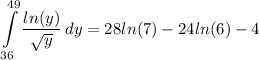
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Integration

Book: College Calculus 10e