Answer:
Option: D is the correct answer.
D) –2x + y ≥ 4
x + y < 2
Explanation:
We are asked to find the linear inequality that represents the following graph.
Clearly from the graph we could observe that one line is dotted and the other is solid line.
i.e. one inequality is strict while the other is a inequality with equality sign.
Dashed line:
It passes through (0,2) and (2,0).
Hence, the equation of line is:
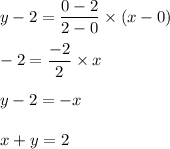
As the shaded region is towards the origin.
Hence, the inequality will be:
x+y<2.
Solid line:
The line passes through (-2,0) and (0,4).
hence equation of line is:
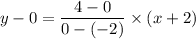
i.e
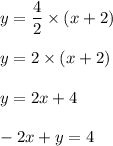
Now, as the shaded region is away from the origin hence the inequality which will be used is:

Hence, option: D is the correct answer.
D. –2x + y ≥ 4
x + y < 2