Question

Jaidee and Eugene are selling fruit for a school fundraiser. Customers can buy small boxes of tangerines and large boxes of tangerines. Jaidee sold 5 small boxes of tangerines and 4 large boxes of tangerines for a total of $130. Eugene sold 2 small boxes of tangerines and 5 large boxes of tangerines for a total of $120. What is the cost each of one small box of tangerines and one large box of tangerines?

Answer 1

This is going to take a while. First, set up equations for both Jaidee and Eugene. Jaidee's equation is
`5s+4l=130` s represents the cost of the small box of tangerines and
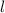
`l` represents the cost of the large box of tangerines. Eugene's equation is
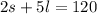
`2s+5l=120` . Next, I'm going to solve the equations through elimination. Multiply -2 by the first equation and multiply 5 by the second equation.
`-2(5s+4l=130)=-10s-8l=-260` Do the same for the second equation.
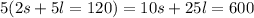
`5(2s+5l=120)=10s+25l=600` . Then add
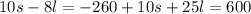
`10s-8l=-260+10s+25l=600` This gives you
`17l=340` . Next divide on both sides
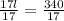
`(17l)/(17) =(340)/(17)` this will give you
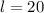
`l=20` . So you know what
`l` is. Next, plug in
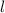
`l` to
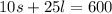
`10s+25l=600` which looks like this
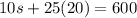
`10s+25(20)=600`. Solve 10s+500=600 = 10s+500-500=600-500 =10s=100 = 10s/10=100/10 = s=10 . So each box of small tangerines costs $10 and each box of large tangerines cost $20.