Question

A farm raises cows and chickens. The farm has total of 43 animals. One day he counts the legs of all his animals and realizes he has a total of 122. How many cows and chickens does he have?

Answer 1

Assume that there are x cows and y chickens in the form

Since there are 43 animals, then

Add x and y, then equate the sum by 43

`x+y=43\rightarrow(1)`

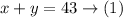
Since a cow has 4 legs and a chicken has 2 legs

Since there are 122 legs, then

Multiply x by 4 and y by 3, then add the products and equate the sum by 122

`4x+2y=122\rightarrow(2)`

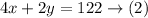
Now, we have a system of equations to solve it

Multiply equation (1) by -2 to make the coefficients of y equal in values and opposite in signs

`\begin{gathered} -2(x)+-2(y)=-2(43) \\ -2x-2y=-86\rightarrow(3) \end{gathered}`

Add equations (2) and (3) to eliminate y

`\begin{gathered} (4x-2x)+(2y-2y)=(122-86) \\ 2x+0=36 \\ 2x=36 \end{gathered}`

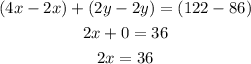
Divide both sides by 2

`\begin{gathered} (2x)/(2)=(36)/(2) \\ x=18 \end{gathered}`

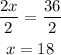
Substitute x by 18 in equation (1)

`18+y=43`

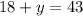
Subtract 18 from each side

`\begin{gathered} 18-18+y=43-18 \\ y=25 \end{gathered}`

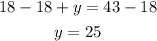
The answer is

There are 18 cows and 25 chickens on the farm