Question

Write this absolute value function as a piecewise function. y= 2|x|-3

Answer 1

A function can be written as piecewise function if it changes its behaviour ( increasing or decreasing ) about a point.

`\begin{gathered} Thefunctionf\mleft(x\mright)=y=|x|changesitsbehaviourat|x|=0ie,x=0. \\ y=|x|=x,ifx<0=-x,ifx>0 \\ |x|=a\Rightarrow x=\pm a \end{gathered}`

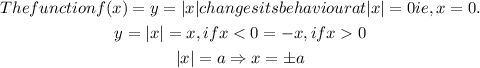
Here Here,

The given function is : y = 2|x| - 3

Here,

The function will change its behaviour when

| x | = 0

=> x = 0

Now,

If x < 0 , then ;

=> y = 2[–(x)] – 3

=> y = –2x – 3

=> y = – 2x – 3

If x ≥ 0 , then ;

=> y = 2(x) – 3

=> y = 2x – 3

=> y = 2x -3

Hence ,

y = – 2x – 3 , if x < 0

y = 2x - 3 , if x ≥ 0

`f(x)=\begin{cases}-2x-3\text{ , if x < }0 \\ \\ 2x-3\text{ , if x }\ge0\end{cases}`