Question

If 10 g of a radioactive substance are present initially and 9 yr later only 5 g remain, how much of the substance will be present after 18 yr?After 18 yr there will be g of a radioactive substance.(Round the final answer to three decimal places as needed. Round all intermediate values to seven decimal places as needed.)

Answer 1

Given:

The initial amount of substance, No=10 g.

The amount of substance left after 9 years, N=5 g.

Since 10 g of substance is present initially, and it became 5 g(half of the initial amount) in 9 years, the half life of the substance is, t =9 years.

Hence, the expression for the amount remaining after T years is,

`N(t)=N_0((1)/(2))^{\frac{T}{t_{}}}`

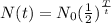
To find the amount of substance remaining after 18 years, put T=18, N0=10 and t=9 in the above equation.

`\begin{gathered} N(18)=10*((1)/(2))^{(18)/(9)} \\ N(18)=10((1)/(2))^2 \\ =(10)/(4) \\ =2.5\text{ g} \end{gathered}`

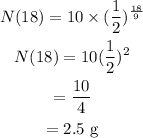
Therefore, after 18 years 2.5 g of the radioactive substance will remain.