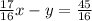Question

Write an equation of the line through (-3,- 6) having slope17/16Give the answer in standard form.The equation of the line is

Answer 1

The equation of a line in Standard form is:

`Ax+By=C`

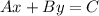
Where "A", "B" and "C" are Integers ("A" is positive).

The Slope-Intercept form of the equation of a line is:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept.

In this case you know that:

`m=(17)/(16)`

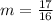
And knowing that the line passes through the point

`\mleft(-3,-6\mright)`

You can substitute values and solve for "b":

`\begin{gathered} y=mx+b \\ -6=((17)/(16))(-3)+b \\ \\ \\ -6=-(51)/(16)+b \\ \\ -6=-(51)/(16)+b \\ \\ -6+(51)/(16)=b \\ \\ b=-(45)/(16) \end{gathered}`

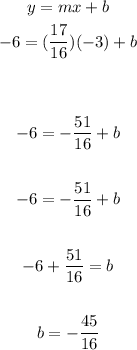
Then, the equation of this line in Slope-Intercept form is:

`y=(17)/(16)x-(45)/(16)`

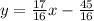
Now that you have this equation, you can write it in Standard form as following:

`\begin{gathered} y+(45)/(16)=(17)/(16)x \\ \\ (45)/(16)=(17)/(16)x-y \\ \\ (17)/(16)x-y=(45)/(16) \end{gathered}`

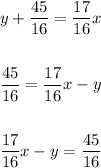
The answer is:

`(17)/(16)x-y=(45)/(16)`