Question

If f(x) is an exponential functionwhere f(-2) = 1 and f(7) = = 63,then find the value of f(1) , to thenearest hundredth.

Answer 1

An exponential function has the form

`y=ab^x`

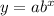
Therefore, to find an exponential function that satisfies our condition, we need to find a and b.

From f(-2) = 1, we have

`1=ab^(-2)\: ^{}\: \: \: \: \; ^{}\: \: \: \: \; (1)`

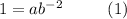
and from f(7) = 63, we have

`63=ab^7\: \: \: \: \; ^{}\: \: \: \: \; (2)`

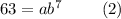
Solving for a in equation (1) gives

`a=b^2`

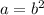
substituting this value of a into equation (2) gives

`63=b^2\cdot b^7`
`63=b^8`
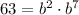
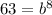
`\begin{gathered} \therefore b=\sqrt[8]{63} \\ b=1.6785 \end{gathered}`

With the value of b in hand, we now find the value of a:

`\begin{gathered} a=b^2 \\ \therefore a=2.8173 \end{gathered}`

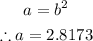
Hence, the exponential function is

`f(x)=(2.8173)(1.6785)^x`

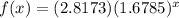
Evaluating the above function at x = 1 gives

`\begin{gathered} f(1)=(2.8173)(1.6785)^1 \\ \boxed{\therefore f(1)=4.73.} \end{gathered}`

which is our answer!