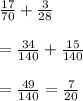Question

Please help #22 please sos

Answer 1

Even with fractions, we still use the order of operations. The acronym to remember this is PEMDAS. First is parentheses, then exponents, multiplication/division, addition/subtraction.

The given problem is
`(1)/(5)( 3(1)/(2)* (4)/(7) - (11)/(14)) + (3)/(28)`.
First we simplify what's in the parentheses. PEMDAS then applies in the parentheses, and since they are no further parentheses or exponents, we look at multiplication/division and multiply. First let's have all improper fractions.

`(1)/(5)( 3(1)/(2)* (4)/(7) - (11)/(14)) + (3)/(28) \\ = (1)/(5)( (7)/(2)* (4)/(7) - (11)/(14)) + (3)/(28)`.

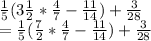
Next multiply the fractions: numerator with numerator and denominator with denominator.

`(1)/(5)( (7)/(2)* (4)/(7) - (11)/(14)) + (3)/(28) \\\\ = (1)/(5)( (28)/(14) - (11)/(14)) + (3)/(28)`

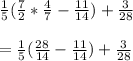
Next in the parentheses is addition/subtraction.

`(1)/(5)( (28)/(14) - (11)/(14)) + (3)/(28) \\\\ = (1)/(5) ( (17)/(14)) + (3)/(28)`

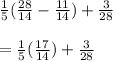
Now apply PEMDAS again, and since there are no parentheses or exponents to simplify, we multiply.

`(1)/(5) ( (17)/(14)) + (3)/(28) \\\\ = (17)/(70) + (3)/(28)`

Finally find a common denominator and add.

`(17)/(70) + (3)/(28) \\\\ = (34)/(140) + (15)/(140) \\\\ = (49)/(140) = (7)/(20)`

Answer 2

1/5( 5/2 x 4/7 -11/7) + 3/28

Multiply the terms (5/2 x 4/7) in bracket then subtract it from 11/14. Result will be 10/7.

Then multiply 1/5 with 10/7. The result will be 2/7.

2/7 + 3/28 = 11/28

T