We have to calculate a 90% confidence interval for the proportion that chose the answer "Spruce".
The sample proportion is p = 0.869:
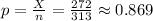
The standard error of the proportion is:
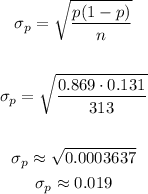
The critical z-value for a 90% confidence interval is z = 1.645.
The margin of error (MOE) can be calculated as:

Then, the lower and upper bounds of the confidence interval are:
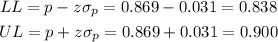
Answer: The 90% confidence interval for the population proportion is (0.838, 0.900).