Question

Use synthetic division to find P (-10) for P(x)=2x^3+14x^2-58x

Answer 1

The polynomial remainder theorem states that the remainder upon dividing a polynomial
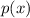
`p(x)` by
`x-c` is the same as the value of
`p(c)`, so to find
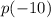
`p(-10)` you need to find the remainder upon dividing


`(2x^3+14x^2-58x)/(x+10)`

You have

..... | 2 ... 14 ... -58
-10 | ... -20 ... 60
--------------------------
..... | 2 ... -6 .... 2

So the quotient and remainder upon dividing is


`(2x^3+14x^2-58x)/(x+10)=2x-6+\frac2{x+10}`

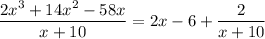
with a remainder of 2, which means
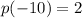
`p(-10)=2`.