Answer:
(i)
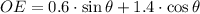 , (ii)
, (ii)
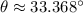 , (iii) The maximum value of OE is approximately 1.523 meters, which is associated with an angle of approximately 23.199º.
, (iii) The maximum value of OE is approximately 1.523 meters, which is associated with an angle of approximately 23.199º.
Explanation:
(i) From Geometry, we get that sum of internal angles of trangle AOD.
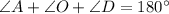 (1)
(1)
If we know that
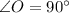 and
and
 , then the value of
, then the value of
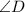 is:
is:
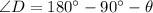
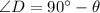 (2)
(2)
But we also have the following identity:
 (3)
(3)
If we know that
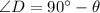 and
and
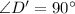 , then the value of
, then the value of
 is:
is:
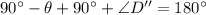
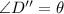 (4)
(4)
By Trigonometry, we derive the following formula:
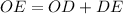
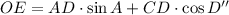 (5)
(5)
If we know that
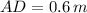 ,
,
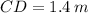 ,
,
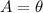 and
and
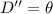 , then the value of OE is:
, then the value of OE is:
 (6)
(6)
(ii) If we know that
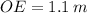 , then the value of
, then the value of
 :
:
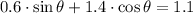
By trial and error, we find that
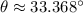 .
.
(iii) Let
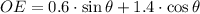 , the first and second derivatives of the function are, respectively:
, the first and second derivatives of the function are, respectively:
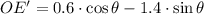 (7)
(7)
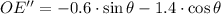 (8)
(8)
We equalize the first derivative of the function to zero and solve for
 :
:
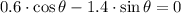
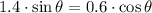
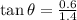
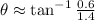
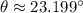
And we evaluate the second derivative:
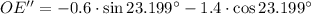
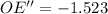
Then, the critical value is associated with an absolute maximum.
The maximum value of OE is:
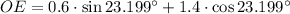
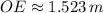
The maximum value of OE is approximately 1.523 meters, which is associated with an angle of approximately 23.199º.