Question

^3 sq root of 1+x+sq root of 1+2x =2

Answer 1

The given equation is

`\sqrt[3]{1+x+√(1+2x)}=2`

First, we need to elevate each side to the third power.

`\begin{gathered} (\sqrt[3]{1+x+√(1+2x)})^3=(2)^3 \\ 1+x+√(1+2x)=8 \end{gathered}`

Second, subtract x and 1 on both sides.

`\begin{gathered} 1+x+√(1+2x)-x-1=8-x-1 \\ √(1+2x)=7-x \end{gathered}`

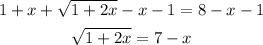
Third, we elevate the equation to the square power to eliminate the root

`\begin{gathered} (√(1+2x))^2=(7-x)^2 \\ 1+2x=(7-x)^2 \end{gathered}`

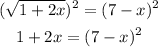
Now, we use the formula to solve the squared binomial.

`(a-b)=a^2-2ab+b^2`
`\begin{gathered} 1+2x=7^2-2(7)(x)+x^2 \\ 1+2x=49-14x+x^2 \end{gathered}`

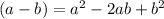
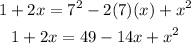
Now, we solve this quadratic equation

`\begin{gathered} 0=49-14x+x^2-2x-1 \\ x^2-16x-48=0 \end{gathered}`

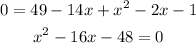
We need to find two number which product is 48 and which difference is 16. Those numbers are 12 and 4, we write them down as factors.

`x^2-16x-48=(x-12)(x+4)`

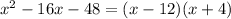
So, the possible solutions are

`\begin{gathered} x-12=0\rightarrow x=12 \\ x+4=0\rightarrow x=-4 \end{gathered}`

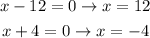
However, we need to verify each solution to ensure that each of them satisfies the given equation. We just need to evaluate it with those two solutions.

`\begin{gathered} \sqrt[3]{1+x+√(1+2x)}=2\rightarrow\sqrt[3]{1+12+√(1+2(12))}=2 \\ \sqrt[3]{13+√(1+24)}=2 \\ \sqrt[3]{13+√(25)}=2 \\ \sqrt[3]{13+5}=2 \\ \sqrt[3]{18}=2 \\ 2.62=2 \end{gathered}`

As you can observe, the solution 12 doesn't satisfy the given equation.

Therefore, the only solution is -4.