Question

Need help asap please!​

Answer 1

The distance of two complex numbers

`z_(1) z_(2) = √(82)` = 9.055

Explanation:

Step(i):-

Given that z₁ = 6-3i and z₂ = -3-4i

We know that 'z' represents a point on the argand plane with absicca 'x' and ordinate 'y'

Now given points are ( x₁ , y₁) = (6 ,-3) and (x₂, y₂) = (-3 , -4)

Step(ii):-

The distance of two points

`\sqrt{(x_(2) -x_(1) )^(2) +(y_(2) - y_(1) )^(2) }`

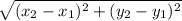
z₁ z₂
`= \sqrt{(-3-6)^(2) +(-4-(-3)^(2) }`

`z_(1) z_(2) = √(81+1)`

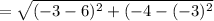
`z_(1) z_(2) = √(82)` =9.055