Question

Help!

What proportional segment lengths verify that BC ∥ DE ?
Enter your answers in the boxes.

Answer 1

4 over 3.2 and 6 over 4.8

Answer 2

`(4)/(10) =(3.2)/(8)`

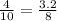
Explanation:

Given : AD=4

DB=6

AE=3.2

EC=4.8

AB=10

AC=8

Solution :

Theorem :A transversal that is parallel to one of the sides in a triangle divides the other two sides proportionally.

So,

`(AD)/(AB) =(AE)/(AC)`

`(4)/(10) =(3.2)/(8)`

`(4)/(10) =(32)/(80)`

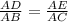
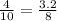
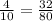
Reduce the fraction both sides by dividing both the fractions with common factor respectively.

`(2)/(5) =(2)/(5)`

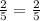
Thus ans in boxes will be
`(4)/(10) =(3.2)/(8)`

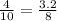
This verify that BC ∥ DE.