Question

The ten students in a club are lined up in a row for a group photograph. How many different arrangements are possible if the club includes one set of identical triplets wearing matching clothes? 604,800 720 10,000,000

Answer 1

It's 10!/3! so its 604, 800.

Answer 2

604800

Explanation:

Given : The ten students in a club are lined up in a row for a group photograph.

To Find: How many different arrangements are possible if the club includes one set of identical triplets wearing matching clothes?

Solution:

The club includes one set of identical triplets wearing matching clothes

So, the remaining students = 10-3 = 7

Now there is an arrangement between these seven students.

Since order has to be maintained .So, we will use permutation

Formula :
`^nP_r=(n!)/((n-r)!)`

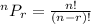
So,
`^(10)P_7=(10!)/((10-7)!)`

`^(10)P_7=(10!)/((3)!)`

`^(10)P_7= 604800`

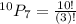
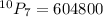
Hence there are 604800 possible arrangements if the club includes one set of identical triplets wearing matching clothes