Question

Determine whether point (4, -3) lies on the line with equation y = -2x + 5 by using substitution and by graphing.

Answer 1

The equation of the line is:

`y=-2x+5`

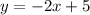
And we need to find if the point (4,-3) lies on the line.

To solve by using substitution, we need to remember that an ordered pair always has the form (x, y) --> the first number is the x-value, and the second number is the y-value.

In this case (4,-3):

x=4

and

y=-3

So now, we substitute the x value into the equation, and if the y-value we get in return is -3 --> the point lies on the line. If not, the point does not lie on the line.

`\begin{gathered} y=-2x+5 \\ \text{Substituting x=4} \\ y=-2(4)+5 \\ y=-8+5 \\ y=-3 \end{gathered}`

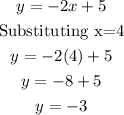
We do get -3 as the y-value which matches with the indicated y value of the point.

Thus, by substitution, we confirm that the point lies on the line.

To check the result by graphing, we need to graph the line. The graph of the line is shown in the following image:

In the image, we can see that the marked point on the line is the point we were looking for (4,-3). So we confirm by the graphing method that the point lies on the line.

Answer: It is confirmed by substitution and graphing that the point (4,-3) lies on the line.