Answer:
The height of the cactus is 13.5 ft.
C is correct.
Explanation:
In the sunlight, a cactus casts a 9 ft shadow.
At the same time an oversized groundhog 6 ft tall casts a 4 ft shadow.
Ratio of shadow to height is same for all at same time.
Ratio of groudhog
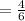
Let the height of the cactus be x ft
Ratio of cactus
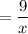
Both ratio must be equation because similar triangle theorem, Ratio of two sides is equal.
Therefore,
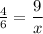
Now we solve for x
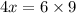
 ft
ft
Hence, The height of the cactus is 13.5 ft.