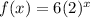The form of the exponential function is
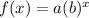
a is the initial value (value f(x) at x = 0)
b is the growth/decay factor
Since the function has points (0, 6) and (3, 48), then
Substitute x by 0 and f(x) by 6 to find the value of a

Substitute the value of a in the equation above
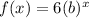
Now, we will use the 2nd point
Substitute x by 3 and f(x) by 48
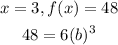
Divide both sides by 6
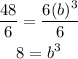
Since 8 = 2 x 2 x 2, then

Change 8 to 2^3
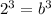
Since the powers are equal then the bases must be equal

Substitute the value of b in the function
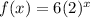
The answer is:
The formula of the exponential function is