Question

What is the solution of the equation 4(3-x)^4/3-5=59

Answer 1

The solution is x=−5

Answer 2

Given the equation:
`4(3-x)^{(4)/(3)} -5 = 59`

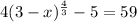
Add 5 to both sides we have;

`4(3-x)^{(4)/(3)} = 64`

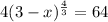
Divide by 4 both sides we have;

`(3-x)^{(4)/(3)} =16`

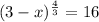
or

`3-x = (16)^{(3)/(4)`

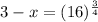
Using exponent rules;

`a^{(n)/(m)} = (\sqrt[m]{a})^n`

then;

`3-x =( \sqrt[4]{16})^3`

Simplify:

`3-x = (2)^3`

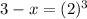
or

`3-x = 8`

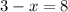
subtract 3 from both sides we have;

`-x = 5`

Divide both sides by -1 we get

`x = -5`

Therefore, the solution for the given equation is, -5