Question

A play court on the school playground is shaped like a square joined by a semicircle. The perimeteraround the entire play court is 182.8 ft., and 62.8 ft. of the total perimeter comes from the semicircle.aWhat is the radius of the semicircle? Use 3.14 for atb.The school wants to cover the play court with sports court flooring. Using 3.14 for, how manysquare feet of flooring does the school need to purchase to cover the play court?

Answer 1

The total perimeter of the court is 182.8 ft, of this, 62.8ft represents the perimeter of the semicircle.

a)

The perimeter of the semicircle is calculated as the circumference of half the circle:

`P=r(\pi+2)`

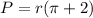
Now write it for r

`\begin{gathered} (P)/(r)=\pi \\ r=(P)/(\pi) \end{gathered}`

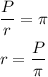
Knowing that P=62.8 and for pi we have to use 3.14

`\begin{gathered} r=(62.8)/(3.14) \\ r=20ft \end{gathered}`

The radius of the semicircle is r=20 ft

b.

To solve this exercise you have to calculate the area of the whole figure.

The figure can be decomposed in a rectangle and a semicircle, calculate the area of both figures and add them to have the total area of the ground.

Semicircle

The area of the semicircle (SC) can be calculated as

`A_(SC)=(\pi r^2)/(2)`

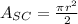
We already know that our semicircla has a radius of 10ft so its area is:

`A_(SC)=(3.14\cdot20^2)/(2)=628ft^2`

Rectangle

To calculate the area of the rectangle (R) you have to calculate its lenght first.

We know that the total perimeter of the court is 182.8ft, from this 62.8ft corresponds to the semicircle, and the rest corresponds to the rectangle, so that:

`\begin{gathered} P_T=P_R+P_(SC) \\ P_R=P_T-P_(SC) \\ P_R=182.8-62.8=120ft \end{gathered}`

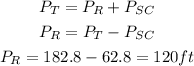
The perimeter of the rectangle can be calculated as

`P_R=2w+2l`

The width of the rectangle has the same length as the diameter of the circle.

So it is

`w=2r=2\cdot20=40ft`

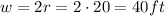
Now we can calculate the length of the rectangle

`\begin{gathered} P_R=2w+2l \\ P_R-2w=2l \\ l=(P_R-2w)/(2) \end{gathered}`

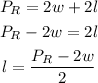
For P=120ft and w=40ft

`\begin{gathered} l=(120-2\cdot40)/(2) \\ l=20ft \end{gathered}`

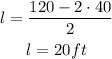
Now calculate the area of the rectangle

`\begin{gathered} A_R=w\cdot l \\ A_R=40\cdot20 \\ A_R=800ft^2 \end{gathered}`

Finally add the areas to determine the total area of the court

`\begin{gathered} A_T=A_(SC)+A_R=628ft^2+800ft^2 \\ A_T=1428ft^2 \end{gathered}`