Question

Write an absolute value inequality that represents all real numbers more than 4 units away from x

Answer 1

We have to write as inequality the following

"All real numbers more than 4 units away from x"

"4 units away from x" means four units plus x. So, the expression would be

`|x|>4`

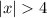
Where x represents real numbers.

This expression is referring to all real numbers more than 4 units and less than -4 units because according to the property of absolute values for inequalities, we have

`|x|>x-4\rightarrow x>x-4,or,x<-(x-4)`

This is represented in the following graph to see it better

For x=1

`\begin{gathered} |1|>x-4\rightarrow1>1-4,or,1<-(1-4) \\ 1>-3 \\ 1<3 \end{gathered}`

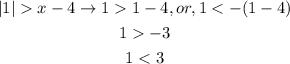
Both results are true.

To find this absolute value inequality we used the following property

`|x|>a\rightarrow a>b,or,a<-b`

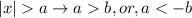
Where the absolute value inequality has "more than" we rewrite the expression in two inequalities.