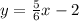Question

what is an equation of the line that passes through the point -6 and -7 and is perpendicular to the line 6x+5y=30I got y=5/6-2 but apparently its wrong

Answer 1

First we can find the slope. The standard form of the equation of a line is:

`y=ax+b`

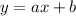
Where a is the slope and b is the intercept.

When 2 lines are perpendicular, the slopes are reciprocal and opposite to each other. If we write the given equation of the perpendicular line in the standard form we have:

`6x+5y=30\rightarrow y=-(6)/(5)x+(30)/(5)\rightarrow y=-(6)/(5)x+6`

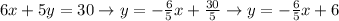
So you got the slope right, it's 5/6.

Now, with the given point we find the intercept. The point is x = -6 and y = -7, so we replace these values into the expression we have until now:

`y=(5)/(6)x+b`
`-7=(5)/(6)(-6)+b`

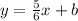
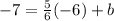
And solve for b

`-7=-5+b\rightarrow b=-7+5=-2`

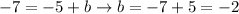
So the equation of the line is:

`y=(5)/(6)x-2`