Question

A beach ball rolls off a cliff and onto the beach. The height, in feet, of the beach ball can be modeled by the function h(t)=64−16t2, where t represents time, in seconds.What is the average rate of change in the height, in feet per second, during the first 1.25 seconds that the beach ball is in the air?Enter your answer as a number, like this: 42

Answer 1

STEP - BY - STEP EXPLANATION

What to find?

The average rate of change in the height, in feet per second, during the first 1.25 seconds that the beach ball is in the air.

Given:

`h(t)=64-16t^2`

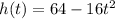
Step 1

Differentiate the heigh with reospect to t.

The rate of change of height is the differentiation of the height.

`(dh(t))/(dt)=-32t`

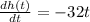
Step 2

Substitute t= 1.25

`h^(\prime)(t)=-32(1.25)`
`=-40ft\text{ /s}`

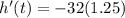
ANSWER

Average rate = -40 ft / s