Question

Which set of numbers could represent the lengths of the sides of a right triangle?

9, 10, 11

16, 32, 36

8, 12, 16

3, 4, 5

Answer 1

3,4,5
using a^2 +b^2 = c^2 plug in any of the numbers i plugged in
3^2 +4^2 which gave me 9+16 and that is 25 and 5^2 is 25

Answer 2

3, 4, 5

Explanation:

We are given the sets of numbers and we are supposed to find Which set of numbers could represent the lengths of the sides of a right triangle

So, we need to use Pythagoras theorem

`Hypotenuse^(2) =Perpendicular^(2) +Base^(2)`

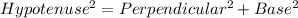
1) 9,10,11

Hypotenuse = 11

So, using Pythagoras theorem

`11^(2) =10^(2) +9^(2)`

`121=100 +81`

`121\\eq 181`

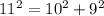
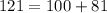
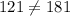
Since the given set does not satisfy the Pythagoras theorem.So, the given set of number could not represent the lengths of the sides of a right triangle.

2)16, 32, 36

Hypotenuse = 36

So, using Pythagoras theorem

`36^(2) =32^(2) +16^(2)`

`1296=1024 +256`

`1296\\eq 1280`

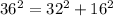
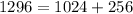
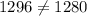
Since the given set does not satisfy the Pythagoras theorem.So, the given set of number could not represent the lengths of the sides of a right triangle.

3)8, 12, 16

Hypotenuse = 36

So, using Pythagoras theorem

`16^(2) =12^(2) +8^(2)`

`256=144 +64`

`256\\eq 208`

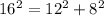
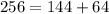
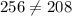
Since the given set does not satisfy the Pythagoras theorem.So, the given set of number could not represent the lengths of the sides of a right triangle.

4)3, 4, 5

Hypotenuse = 5

So, using Pythagoras theorem

`5^(2) =4^(2) +3^(2)`

`25=16 +9`

`25\=25`

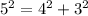
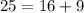
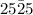
Since the given set satisfy the Pythagoras theorem.So, the given set of number could represent the lengths of the sides of a right triangle.

Hence 3, 4, 5 is the set of numbers could represent the lengths of the sides of a right triangle