I assume by smallest number, you're really looking for the smallest *positive* number.
Notice that -5 fits all these requirements:
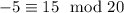
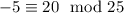
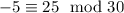


Any number of the form
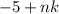
will also satisfy these conditions, where
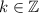
and

is the least common multiple of the moduli. You have
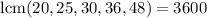
so the least positive number would be achieved with
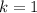
, giving 3595 as the answer. (Verified with a script.)