Question

A support cable connects the top of a bridge tower to the road below. The cable is 245.9 feet long and connects to the road at a point 200.7 feet away from the center of the bridge tower. To the nearest tenth of a foot, how tall is the tower? A.317.4 ft B.142.1 ft C.446.6 ft D.246.3 ft

Answer 1

Your answer is 142.1 I took the quiz

Answer 2

Answer: B. 142.1 ft

Explanation:

Given: A support cable connects the top of a bridge tower to the road.

Since, the bridge tower is standing vertical to the the road, therefore, the angle made by it is a right angle.

Therefore, the triangle made by cable(hypotenuse) and bridge tower (Perpendicular) on road (base) must be a right angled triangle.

Therefore, by Pythagoras theorem, we have

`H^2=P^2+B^2\\\\\Rightarrow(245.9)^2=P^2+(200.7)^2\\\\\Rightarrow P^2=(245.9)^2-200.7)^2\\\\\Rightarrow P^2=60466.81-40280.49\\\\\Rightarrow P^2=20186.32\\\\\Rightarrow P=√(20186.32)\\\\\Rightarrow P=142.078569813\approx142.1\ ft`

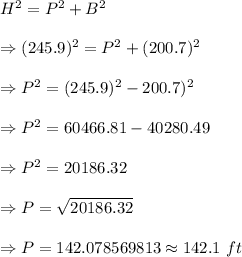
Hence, the height of the bridge tower = 142.1 ft.