Question

Toaster uses a nichrome heating coil and operates at 120 V. When the toaster is turned on at 20°C, the current in the cold coil is 1.5 A. When the coil warms up, the current has a value of 1.3 A. If the thermal coefficient of resistivity for nichrome is 4.5x10-4 1/Co, what is the temperature of the coil?Group of answer choices68oC490oC160oC360oC260oC

Answer 1

Given that the operating voltage is V = 120 V.

The initial temperature of the toaster is T1 = 20 degrees Celsius

The initial current in the coil is I1 = 1.5 A

The final current in the coil is I2 = 1.3 A

The thermal coefficient of resistivity for nichrome is

`\alpha=4.5*10^(-4)^{}\text{ }^(\circ)C^(-1)`

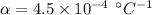
We have to find the final temperature of the coil, T2.

The initial resistance of the coil is

`\begin{gathered} R1=(V)/(I1) \\ =(120)/(1.5) \\ =80\Omega \end{gathered}`

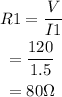
The final resistance of the coil is

`\begin{gathered} R2\text{ =}(V)/(I2) \\ =(120)/(1.3) \\ =92.307\Omega \end{gathered}`

The formula to calculate the final temperature of the coil is

`\begin{gathered} \alpha=((R2-R1))/(R1(T2-T1)) \\ T2-T1=((R2-R1))/(\alpha* R1) \\ T2=((R2-R1))/(\alpha* R1)+T1 \end{gathered}`

Substituting the values, the final temperature will be

`\begin{gathered} T2=\text{ }(92.307-80)/(4.5*10^(-4)*80)+20 \\ \approx360^(\circ)\text{ C} \end{gathered}`

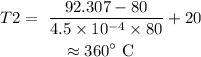
Thus, the final temperature is 360 degrees Celsius.