Question

A training field is formed by joining a rectangle and two semicircles, as shown below. The rectangle is 96 m long and 64 m wide. Find the area of the training field. Use the value 3.14 for n, and do not round your answer. Be sure to include the correct unit in your answer.

Answer 1

To find:

The area of the training field.

Solution:

The training field is made of two semicircles and a rectangle.

The length and width of the rectangle is 96 m and 64 m. So, the area of the rectangle is:

`\begin{gathered} A=l* w \\ =96*64 \\ =6144\text{ m}^2 \end{gathered}`

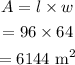
The diameter of the semicircle is 64 m. SO, the radius of the semicircle is 32 m.

The area of two semicircles is:

`\begin{gathered} A=2*(1)/(2)\pi r^2 \\ =3.14*(32)^2 \\ =3.14*1024 \\ =3215.36 \end{gathered}`

So, the area of the training field is:

`\begin{gathered} A=6144+3215.36 \\ =9359.36 \end{gathered}`

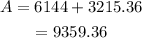
Thus, the area of the training field is 9359.36 m^2.