Answer:
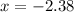

Explanation:
The question is

We let
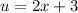 , so the equation becomes:
, so the equation becomes:
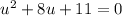
Where
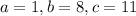
Putting it in the quadratic formula, we have:
Quadratic formula:
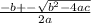
Substituting we have:

We let
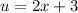 , so x is:
, so x is:

and
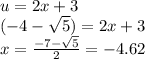
The solutions of the equation is
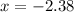 (rounded to 2 decimal places), and
(rounded to 2 decimal places), and
 (rounded to 2 decimal places)
(rounded to 2 decimal places)