Question

A sample of rock is found to contain 200 grams of a parent isotope. How many grams of the parent isotope will remain after one half-life?

Answer 1

100g of isotope, as one half of the isotope will be lost every half life (hence the name).

Answer 2

Answer : The parent isotope remain after one half life will be, 100 grams.

Explanation : Given,

Initial mass of the isotope = 200 g

Time taken by the sample, t =
`t_{(1)/(2)}`

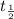
Formula used :

`N=N_o* e^(-\lambda t)\\\\\lambda =\frac{0.693}{t_{(1)/(2)}}`

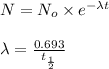
where,

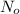`N_o` = initial mass of isotope

N = mass of the parent isotope left after the time, (t)

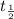`t_{(1)/(2)}` = half life of the isotope

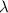`\lambda` = rate constant

`N=N_o* e^{-((0.693)/(t_(1/2)))* t_(1/2)}\\\\N=N_o* e^(-0.693)`

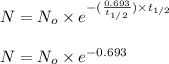
Now put all the given values in this formula, we get

`N=200g* e^(-0.693)`

`N=100g`

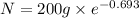
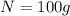
Therefore, the parent isotope remain after one half life will be, 100 grams.