Answer : The volume of helium when the balloon rises to an altitude are, 232 liters.
Explanation :
According to the Boyle's law, the pressure of the gas is inversely proportional to the volume of gas at constant temperature of the gas and the number of moles of gas.

or,
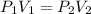
where,
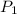 = initial pressure of gas = 203 kPa
= initial pressure of gas = 203 kPa
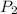 = final pressure of gas = ?
= final pressure of gas = ?
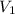 = initial volume of gas = 40 L
= initial volume of gas = 40 L
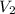 = final volume of gas = 35 L
= final volume of gas = 35 L
Now put all the given values in the above formula, we get the final volume of gas.
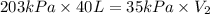

Therefore, the volume of helium when the balloon rises to an altitude are, 232 liters.