Question

Monica wants to measure the dimensions of her rectangular lawn. If the longer side of the lawn is (x + 3) feet and the diagonal length is (x + 4) feet, which function can be used to find the shorter side of her lawn?

Answer 1

This answer is listed above. Square root 2x + 7.

I used it and got it right for the lesson

Answer 2

`\text{Hence, The shortest side of her Lawn is} √(2x+7)\text{ feet}`

Explanation:

Given: Monica wants to measure the dimensions of her rectangular lawn.

If the longer side of the lawn is BC=(x + 3) feet.

If the diagonal length is AC=(x + 4) feet.

Let the shortest side of lawn is AB=y feet

The angle of rectangle is right angle.

Using diagonal, shortest and longer side to make a right angle triangle whose hypotenuse is length of diagonal.

Using Pythagoreous theorem,

`AB^2+BC^2=AC^2`

`y^2+(x+3)^2=(x+4)^2`

`y^2=(x+4)^2-(x+3)^2`

`y^2=x^2+16+8x-x^2-9-6x`

`y^2=2x+7`

`y=\pm √(2x+7)`

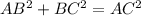
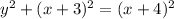
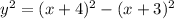
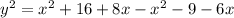
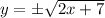
We will ignore the negative value because side of rectangle can't be negative.

`y=√(2x+7)\text{ feet}`

`\text{Hence, The shortest side of her Lawn is} √(2x+7)\text{ feet}`