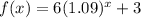Question

Wendy initially has 6 hours of pop music and 3 hours of classical music in her collection. Every month onwards, the hours of pop music in her collection is 9% more than what she had the previous month. Her classical music does not change. Which function shows the total hours of music she will have in her collection after x months?

f(x) = 6(1.09)x + 3
f(x) = 3(1.09)x + 6
f(x) = 6(0.09)x + 3
f(x) = 3(0.09)x+ 6

Answer 1

The correct answer is A
f(x) = 6(1.09)x + 3

Answer 2

f(x) = 6(1.09)x + 3

Step-by-step explanation

To find our exponential equation, we are going to complete the standard exponential growth equation:

`f(x)=a(1+b)^x`

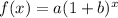
where

`a` is the initial quantity

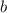`b` is the growth rate in decimal form

`x` is the time (in months for our model)

Since the hours of classical music remain constant, we just need to add them at the end of our calculations.

We know that Wendy initially has 6 hours of pop music, so
`a=6`. We also know that he hours of pop music in her collection is 9% more than what she had the previous month, so
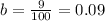`b=(9)/(100) =0.09`. Let's replace the values in our function:

`f(x)=a(1+b)^x`

`f(x)=6(1+0.09)^x`

`f(x)=6(1.09)^x`

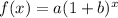
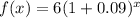
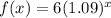
Now we just need to add the hours of classical music to complete our model:

`f(x)=6(1.09)^x+3`