Question

A numbers game run by many state governments allows a player to select a ​three-digit number from 000 to 999. There are 1000 such numbers. A bet of ​$5 is placed on a number. If the number is​ selected, the player wins $500. If any other number is​ selected, the player wins nothing. Find the expected value for the game.

Answer 1

The expected value for the game is of -$4.5

Explanation:

The expected value of the game is given by each scenario multiplied by its probability.

Scenario 1: Player wins

1 correct number from a set of 1000, so probability of 1/1000.

In this case, the player spent $5, but earns $500, so a net of 500 - 5 = $495

Scenario 2: Player loses

Probability of 999/1000.

In this case, the player loses $5.

So, the expected value for the game is:

`E = (495*1)/(1000) - (5*999)/(1000) = -4.5`

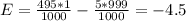
The expected value for the game is of -$4.5