Answer:
The new height of the water will:

Explanation:
The submerged volume due to an object in a liquid, water in our case, is equal to the displaced volume of the water, it is the Archimedes principle.
The volume of a golf ball (sphere) will be
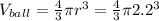

We have 5 golf balls, so the total volume will be:

Using Archimedes' principle the extra volume of water will be 223 cm³
Now, we know that the cylinder has a radius of 3 cm, then the height related to 223 cm³ will be:
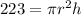

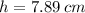
Therefore, the new height of the water will:

I hope it helps you!