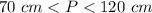we know that
The Triangle Inequality Theorem states that the sum of any 2 sides of a triangle must be greater than the measure of the third side
so
Let
s------> the length of the third side
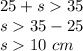
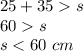
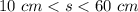
therefore
The answer part a) is
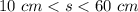
we know that
the perimeter of a triangle is the sum of the length sides
In this problem

For
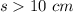
the perimeter is equal to

For

the perimeter is equal to
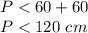
so
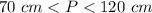
therefore
the answer part b) is