Question

Given the system of constraints name all vertices. Then find the maximum value of the given objective

x≥0
y≥0
y≤ 1/3x+1
5≥y+x
Objective function C = 7x-2y​

Answer 1

`35`

Explanation:

The constraints are

The red line represents the function

`y\leq (1)/(3)x+1`

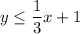
At
`y=0`

`0=(1)/(3)x+1\\\Rightarrow -1=(1)/(3)x\\\Rightarrow x=-3`

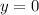
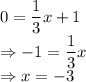
At
`x=0`

`y=0+1\\\Rightarrow y=1`

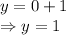
Two points are
`(-3,0),(0,1)`

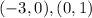
The blue line represents the function

`5\geq y+x`

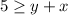
at
`y=0`

`5=x`

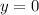
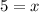
at
`x=0`

`y=5`

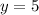
Two points are
`(5,0),(0,5)`

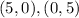
The other two constraints are
`x\geq 0`,
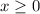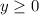`y\geq 0`. So, the point has to be in the first quadrant

From the graph it can be seen there are two points where the function will be maximum let us check them.

`(3,2)`

`7x-2y=7* 3-2* 2=17`

`(5,0)`

`7x-2y=7* 5-2* 0=35`

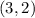
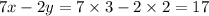
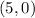
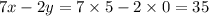
So, the maximum value of the function is
`35`.