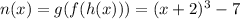Question

Given the functions: f(x) = x^3, g(x) = x - 7, h(x) = x + 2, which composition of functions would

give you the new function n(x) = (x + 2)^3– 7?


A. n(x) = f(g(h(x)))

B. n(x) = g(f(h(x)))

C. n(x) = g(h(f(x)))

D. n(x) = h(f(g(x)))

E. n(x) = f(h(g(x)))

please help a girl is struggling❤️

Answer 1

n(x) = g(f(h(x)))

Explanation:

Given

`f(x) = x^3`

`g(x) = x-7`

`h(x) = x + 2`

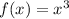
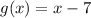
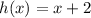
Required

Which function represents
`n(x) = (x + 2)^3 - 7`

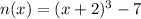
Start by solving for f(h(x)):

Given that:

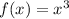`f(x) = x^3` and
`h(x) = x + 2`

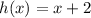
Substitute x + 2 for the x in f(x)

`f(h(x)) = (x + 2)^3`

Next, solve for g(f(h(x)))

Given that:

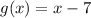`g(x) = x-7` and
`f(h(x)) = (x + 2)^3`

Substitute (x + 2)^3 for the x in g(x)

`g(f(h(x))) = (x + 2)^3 - 7`

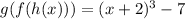
Recall that:

`n(x) = (x + 2)^3 - 7`

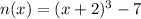
Hence:

`n(x) = g(f(h(x))) = (x + 2)^3 - 7`