Question

Choose the graph that matches the following system of equations:
x + y = 4
2x + 3y = 18

Answer 1

The equations become y=-x+4 and y=-⅔+18. Because of this, I think the graph is the one in the first attachment and the top graph.

Answer 2

Graph A is correct.

Explanation:

We are given the system of inequality,

`x+y=4\\\\2x+3y=18`

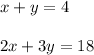
Now, it is required to find the graph of the system of equations.

We have the equations,

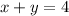`x + y = 4` ............(1)

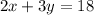`2x + 3y = 18` .............(2)

Multiplying (1) by 2 and subtracting the equations gives us,

`2x+2y-2x-3y=8-18\\-y=-10\\y=10`

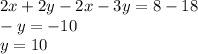
Substituting the value of y in any equation, we get,

`x + 10 = 4\\x=4-10\\x=-6`

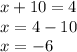
Thus, the solution of the system of equations is (-6,10).

As we have that the point (-6,10) will lie in the second quadrant.

So, the graphs of the equations must intersect in the second quadrant.

Moreover, the slope intercept forms of the equations are given by,

`y=-x+4`

`y =(-2)/(3)x+6`

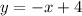
Thus, the slope of the equations are -1 and
`(-2)/(3)`.

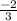Then, the graphs of the equations must be decreasing.

Hence, the correct option is 'Graph A' given below.