Answer:
=5(18^5-1)](https://img.qammunity.org/2018/formulas/mathematics/high-school/uen1sxk97iy9x68emcnchbfhuujsgz6pou.png)
Explanation:
f(x) = x^5 – 1, g(x) = 5x^2, and h(x) = 2x
[g o f o h](9)=g(f(h(9))
LEts fnd h(9) first
h(x)= 2x
h(9)= 2(9) =18
Now plug in 18 for h(9)
[g o f o h](9)=g(f(h(9)) = g(f(18))
now we find f(18)
f(x) = x^5 – 1, f(18)= 18^5 -1
Now we plug in 18^5 -1 for f(18)
[g o f o h](9)=g(f(h(9)) = g(18^5 -1)
Plug it in g(x)
g(x)= 5x^2
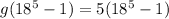
=5(18^5-1)](https://img.qammunity.org/2018/formulas/mathematics/high-school/uen1sxk97iy9x68emcnchbfhuujsgz6pou.png)