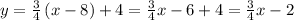Question

Given the equation y − 4 = three fourths(x + 8) in point-slope form, identify the equation of the same line in standard form.

−three fourthsx + y = 10
3x − 4y = −40
y = three fourthsx + 12
y = three fourthsx + 10

Answer 1

You are given the equation


`y-4=(3)/(4)\left(x+8\right)`

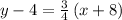
What this represents is the form


`\left(y-y_0\right)=m\left(x-x_0\right)`

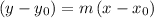
so you can immediately read (although you are not necessarily interested in them) the y-intercept and the x-intercept. The standard form for a linear function is


`y=mx+b`

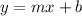
So all you have to do to convert forms is to add the y-intercept to both sides and then simplify. In this case,


`y=(3)/(4)\left(x-8\right)+4=(3)/(4)x-6+4=(3)/(4)x-2`