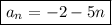Question

Nth term of -7 -12 -17 -22 -27

Answer 1

`a_n=-2-5n`

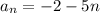
Explanation:

Arithmetic Sequences

The arithmetic sequences are those where any term n is obtained by adding or subtracting a fixed number to the previous term. That number is called the common difference.

The equation to calculate the nth term of an arithmetic sequence is:

`a_n=a_1+(n-1)r`

Where

an = nth term

a1 = first term

r = common difference

n = number of the term

We are given the sequence

-7 -12 -17 -22 -27 ...

The first term of this sequence is a1=-7. We can calculate the common difference by subtracting two consecutive terms:

r = -12 - (-7) = -12 + 7 = -5

The nth term is:

`a_n=-7+(n-1)(-5)`

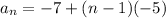
Multiplying:

`a_n=-7-5n+5`

Simplifying:

`\boxed{a_n=-2-5n}`