Answer: The correct answer is Option d.
Explanation:
Formula for Hooke's Law states that:
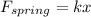
where, F = Force applied = 2N
k = spring constant = 4 N/cm
x = displacement of the spring from equilibrium position = ? cm
Putting values in above equation, we calculate the displacement or distance of the spring, we get:
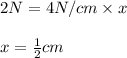
Hence, the correct option is Option d.