Calling x and y the two sizes of the rectangular field, the problem consists in finding the minimum values of x and y that give an area of

.
The area is the product between the two sizes:
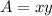
(1)
While the perimeter is twice the sum of the two sizes:
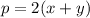
(2)
From (1) we can write
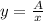
and we can substitute it into (2):
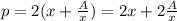
To find the minimum value of the perimeter, we have to calculate its derivative and put it equal to zero:

The derivative of the perimeter is
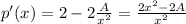
If we require p'(x)=0, we find
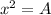
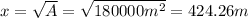
And the other side is
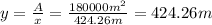
This means that the dimensions that require the minimum amoutn of fencing are (424.26 m, 424.26 m), so it corresponds to a square field.