Answer:
The value of angle α is 18 degree.
Explanation:
Given information: Angles α and β are the two acute angles , α < β.
Given equation is
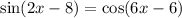
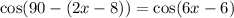
![[\because \sin (90-x)=\cos x]](https://img.qammunity.org/2019/formulas/mathematics/high-school/t5gj457dfzwcvn1zblpgilyj26d1lz1qza.png)
Equating both sides.
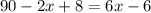
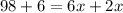
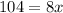

The value of x is 13.
The measure of angles is
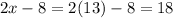
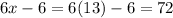
Since 18<72, therefore the value of angle α is 18 degree.