Question

What is the probability that at least 2 students in a class of 36 have the same birthday?

Answer 1

Given a group of n students, and given the fact that there are 365 days in one year, the probability that there are no students with the same birthday is given by

`p_(no) (n) = (364)/(365) \cdot (363)/(365) \cdot ... \cdot (365-n+1)/(365) = (364!)/(365^(n-1) (365-n)!)`
Therefore, the probabilty that there are at least 2 students with same birthday is the complementary of this value:

`p_(yes) (n) = 1 -p_(no)(n) =1- (364!)/(365^(n-1) (365-n)!)`

And if we use n=36 (number of students), we find

`p=1- (364!)/(365^(36-1) (365-36)!) \sim 0.83`
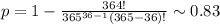
So, the probability that there are at least 2 students in a group of 36 with same birthday is approximately 83%.