Answer:
Amplitude : 4
Period: 2π
Mid-line: y=-1
A is correct
Explanation:
We are given graph of periodic function.
We need to find amplitude, period and midline of the function.
From graph:
Maximum value = 3
Minimum value = -5
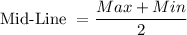
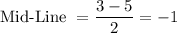
The mid-line equation, y=-1
Amplitude: Distance between mid-line and maximum value
Amplitude = 3-(-1) = 4
The amplitude of given graph is 4
Period: It is time when graph repeat their path.
In the given graph repeat their in 2π of interval.
The period of graph is 2π