Question

Why is the answer C? HELPPPPPPPPPPPPP

Answer 1

I'm not sure how you learned this because there are many different ways of solving it! I'll be using an equation (or you can use Jack's method!). For smaller periods of time it might just be easier to multiply over and over again, but here's the equation I learned for exponential decay problems:

`A = A_0(1-d)^t`

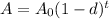
1) A is your final value of what you're measuring, aka final grams of the element in this problem. This is what we're trying to find in this problem!
2)
`A_0` if your initial value of what you're measuring, aka initial grams of the element in this problem. We're told this is 500g.
3) d is the rate of decay in decimal form, which is 8% = 0.08 in this problem.
4) t is the number of times it decays over the time you're given/the number of periods. We're told it decays every 5 years (the period) and want to find out how much is left after 30 years, making the number of periods/times decayed: t = 30/5 = 6.

Plug
`A_0` = 500, d = 0.08, and t = 6 into the equation and solve for A, your final value.

`A = A_0(1-d)^t\\ A = 500(1-0.08)^6\\ A = 303.178 \approx 303`

----

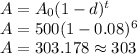
Answer: C

Answer 2

You multiply the initial amount by .92 because that is 92% (100-8) as a decimal. You multiply that answer by .92 and continue to do this 4 more times or as many as many cycles as you are told to do. There may be a faster way but this seems to me to be the easiest